Based on the concept of superposition, the force exerted on a single charge by another charge remains unaffected by the presence of additional charges. The force between charges can be computed individually for each pair, followed by the vector summation of these forces or determination of the net force acting on a specific charge.

The diagram illustrates the interaction between a charge denoted as q1 and additional charges. Therefore, in order to determine the force acting on q1, it is necessary to compute the individual forces exerted by each of the remaining charges, one at a time. The net force, denoted as Vector F1, acting on q1 can be determined by calculating the vector sum.  The vector F1 can be expressed as the sum of vector F12, vector F13, and vector F14. Vector F12 represents the force exerted on charge q1 by charge q2, whereas vector F13 represents the force exerted on charge q1 by charge q3, and vector F14 represents the force exerted on charge q1 by charge q4.

Question: Two identical small charged sphere, each having a mass of 3.0 × 10^–2 kg, hang in equilibrium as shown below. If the length of each string is m and the angle θ = 45°, find the magnitude of the charge on each sphere in nC. (g = 10 m/s²)

Solution:From the right angled triangle, we have sin θ= or, a = L sin θ = (15 m) sin 5° = 0.013 m

Hence, the separation of the spheres is 2a = 0.026 m

                              F.B.D. of one of the spheres:-

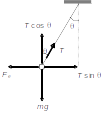
                              Since the sphere is in equilibrium, the resultants of the forces in the horizontal and vertical directions must separately add up to zero. thus

                                         T sin θ – F_e = 0 

                              ⇒           T sin θ = F_e            … (i) 

                              and         T cosθ – mg = 0                

⇒           T cos θ = mg        … (ii) 

                              Dividing equation (i) by equation (ii) , we get

tan θ =   or,  F_e = mg tan θ   

           = (3 × 10^-2 kg) × (10 m/s²) (tan 45°)

                                                                  = 0.3  N  

                              Let q be charge on each sphere.

                              According to Coulomb’s law

                              q  = 15 μC

Question:  Three charges lie along the x-axis as shown in the figure. The positive charge q₁ = 15.0 μC is at x = 2.0 m, and the positive charge q₂ = 6.00 μC is at the origin. Where must a negative charge q₃ be placed on the x-axis such that the resultant force on it is zero? (in mm)   

Solution:Since q₃ is negative and both q₁ and q₂ are positive, the forces and are both attractive. Let x be the co-ordinate of q₃

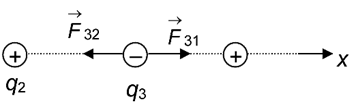
We have

F₃₁ = ; F₃₂ =

                               Since the net force on the change q₃ is zero,

                               we have,  =

                               or,         (4 – 4x + x²) (6 × 10^–6C) = x² (15 × 10^–6 C) 

                              Solving this quadratic equation for x, we get x = 775     

Question:            The electron and the proton in a hydrogen atom are 0.53 × 10^–11 m apart. Compare the electrostatic and the gravitational forces between them in power of 10^–41.

Solution:             The magnitude of the electrostatic force is

                                             F_E =  

                                                     =

                                                     = 8.2 × 10^–8 N 

                              The magnitude of the gravitational force is

                                                     =

                                                      = 3.6 × 10^–47 N

                              The ratio of the forces                                            

 = 44

The  is extremely small. So when we deal with the electrical interaction between elementary particles, gravity may safely be ignored.